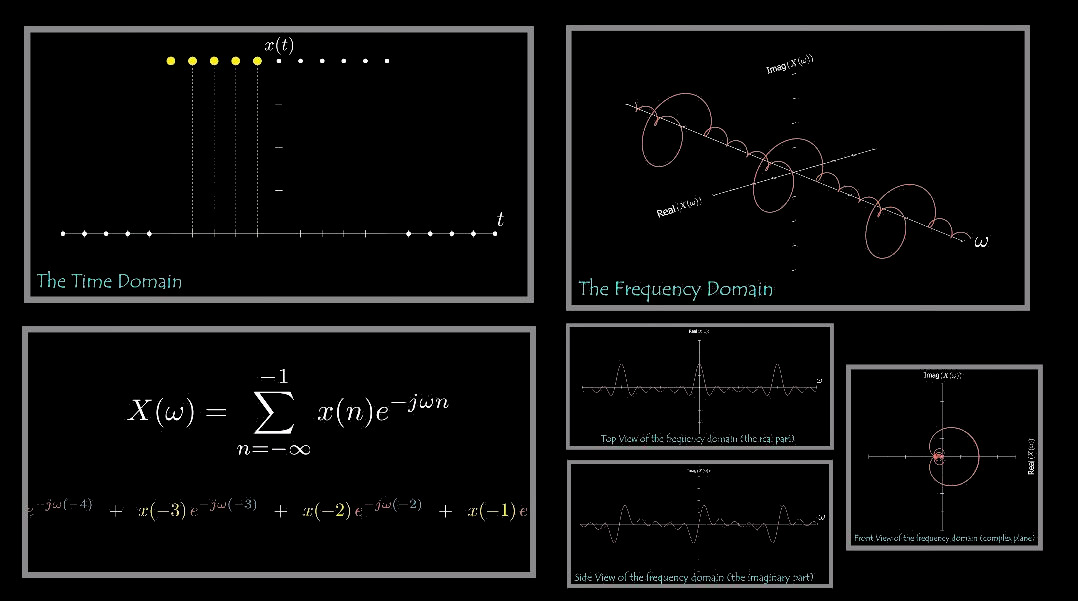
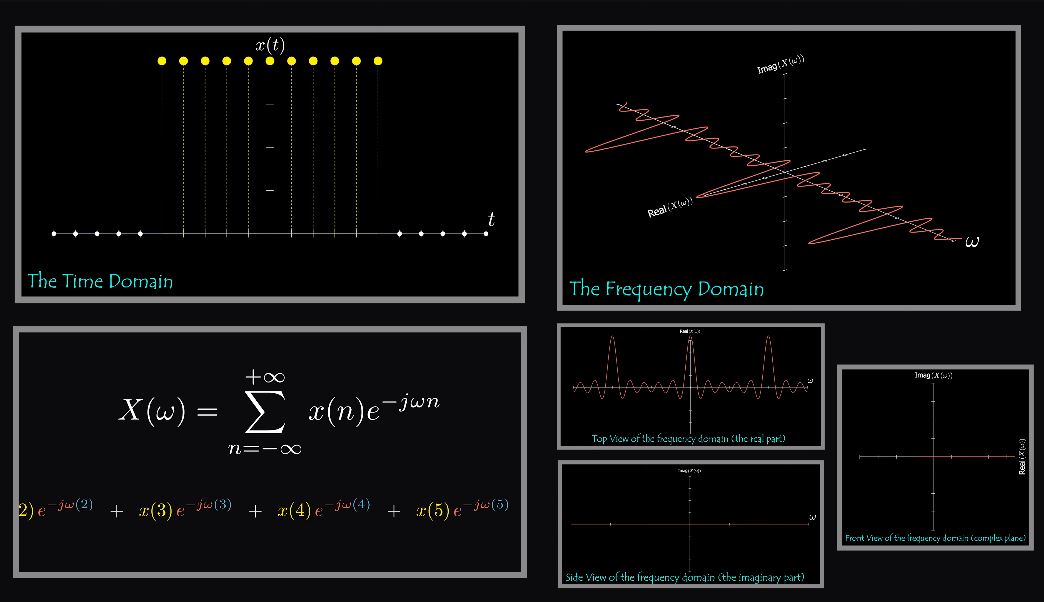
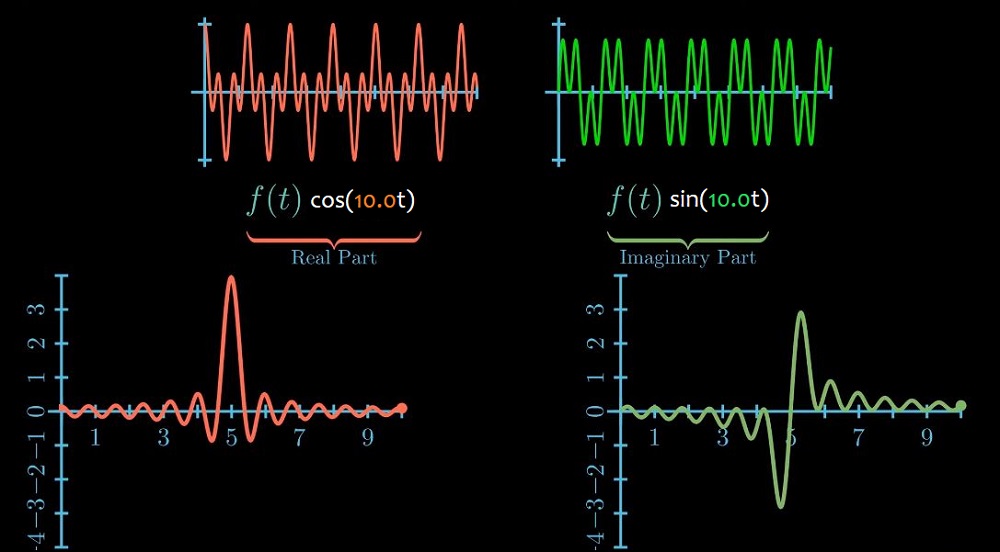
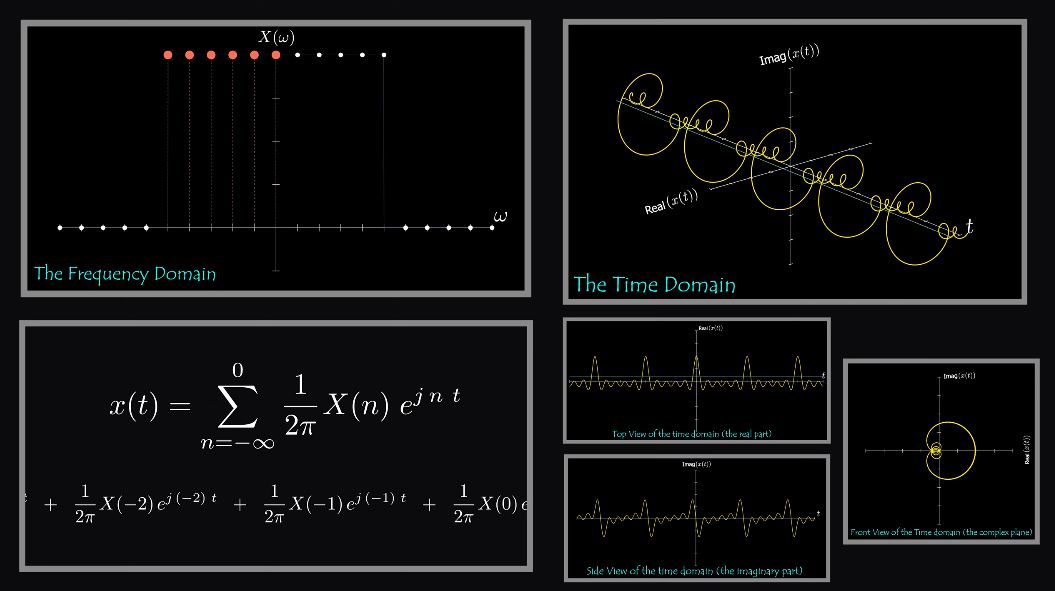
Mathematics Yoshio Sep 8th, 2023 at 8:00 PM 8 0
泰勒展開式(Taylor Expansion)
泰勒級數 Taylor SeriesTaylor Series
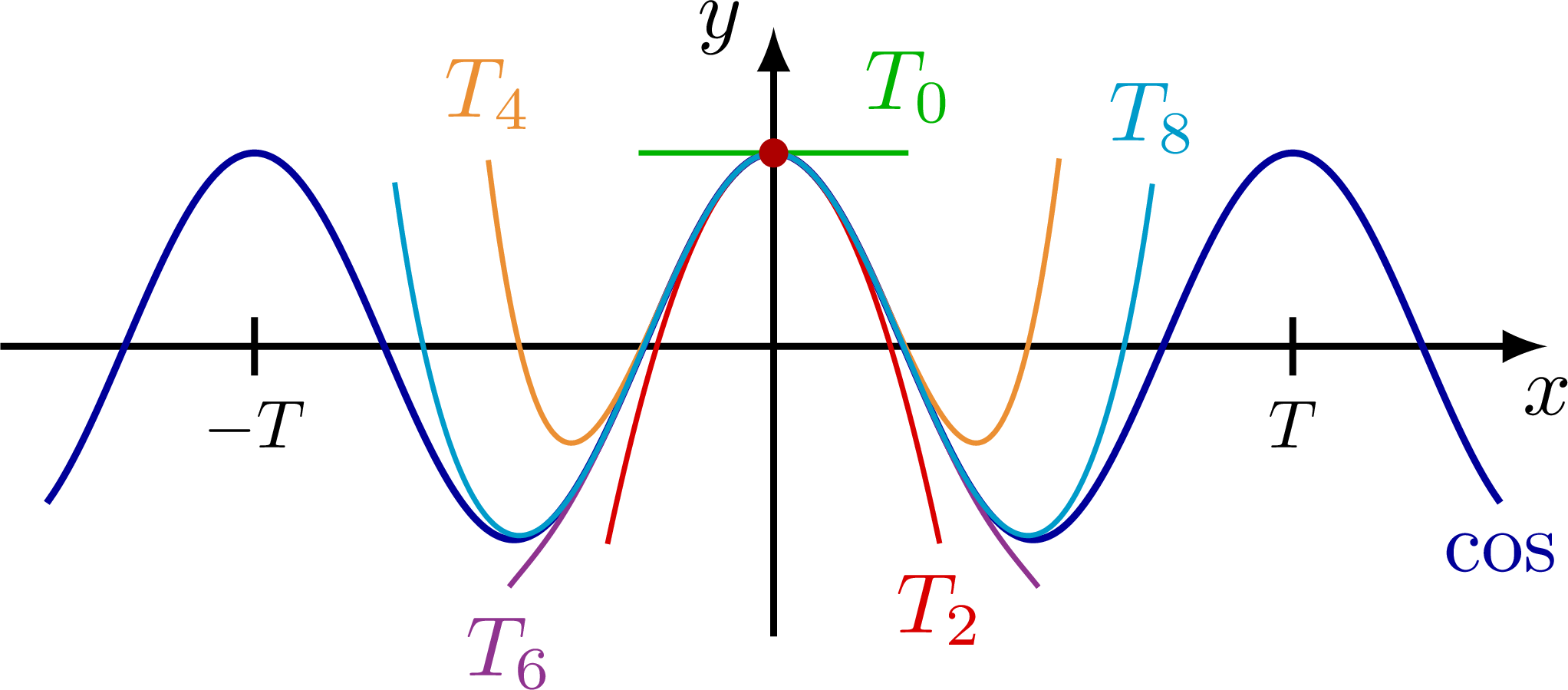
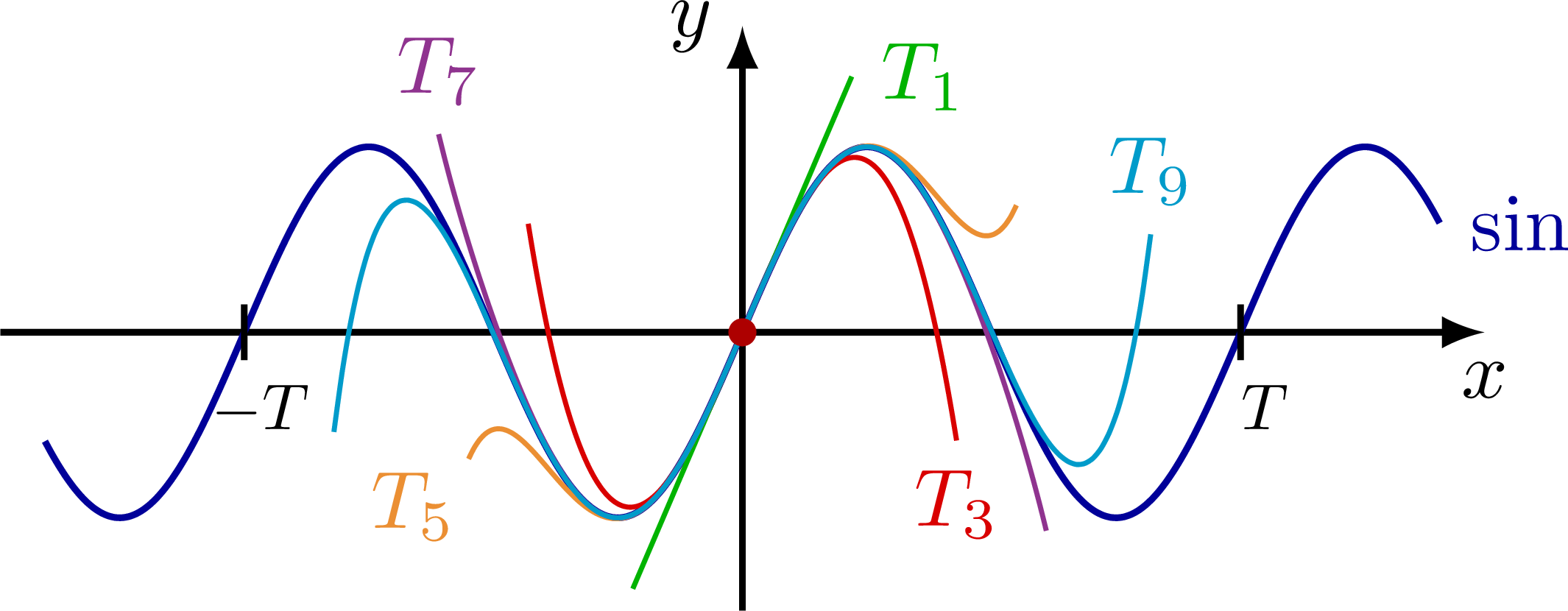
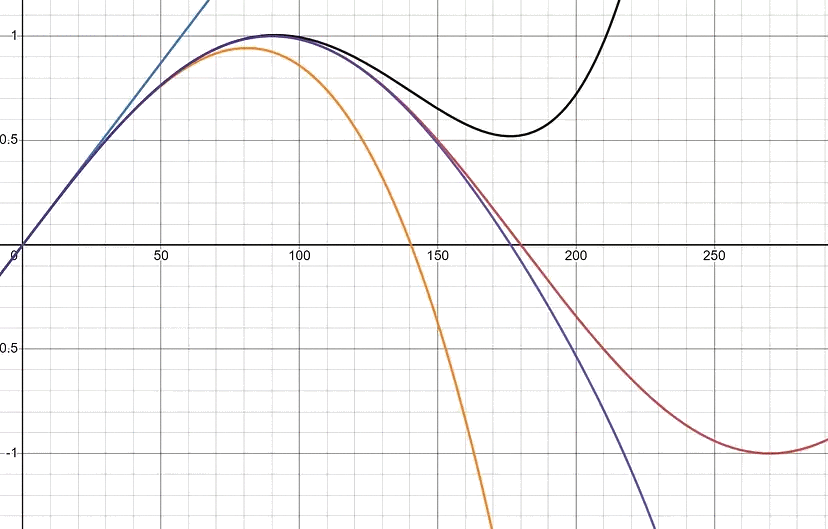
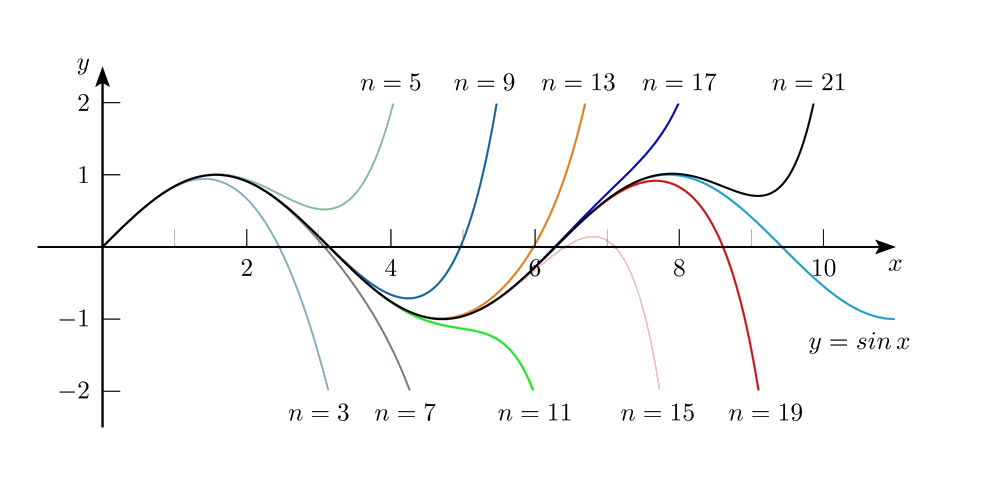
A Taylor series is a series expansion of a function about a point. A one-dimensional Taylor series is an expansion of a real function \(f(x)\) about a point \(x=a\) is given by
\[f(x)=f(a)+f'(a)(x-a)+\frac{f''(a)}{2!}(x-a)^2+\frac{f^{(3)}(a)}{3!}(x-a)^3+\cdots+\frac{f^{(n)}(a)}{n!}(x-a)^n\]
where \(n!\) denotes the factorial of \(f(n)\). In the more compact sigma notation, this can be written as
\[\sum_{n=0}^\infty\frac{f^{(n)}(a)}{n!}(x-a)^n\]
In mathematics, a Power Series is an infinite series of the form
\[f(x)=a_0+a_1(x\;-\;c)+a_2{(x\;-\;c)}^2+...+a_n{(x\;-\;c)}^n=\sum_{n=0}^\infty a_n(x\;-\;c)^n\]
When the center of the series is equal to zero, it is called by Maclaurin Series and takes the simpler form
\[f(x)=a_0+a_1x+a_2x^2+...+a_nx^n=\sum_{n=0}^\infty a_nx^n\]
A polynomial in a single indeterminate x can always be written in the form
\[f(x) = c_0 + c_1 x + c_2 x^2 + ...+ c_k x^k+...=\sum_{k=0}^\infty c_k x^k\]
\(f^{'}(x)=\frac{df(x)}{dx}=c_1+c_2\cdot2\cdot x+c_3\cdot3\cdot x^2+c_4\cdot4\cdot x^3+...\)
\(f^{''}(x)=\frac{df^{'}(x)}{dx}=c_2\cdot2\cdot1+c_3\cdot3\cdot2\cdot x+c_4\cdot4\cdot3\cdot x^2+...\)
\(f^{'''}(x)=\frac{df^{''}(x)}{dx}=c_3\cdot3\cdot2\cdot1+c_4\cdot4\cdot3\cdot2\cdot x+...\)
\(f^{(k)}(x)=\frac{df^{(k-1)}(x)}{dx}=c_k\cdot k!+c_{k+1}\cdot(k+1)!\cdot x+...\)
\(c_k = \frac{f^{(k)}(0)}{k!}\)
\[f(x)=f(0)+\frac{f'(0)}{1!}x+...+\frac{f^{(k)}(0)}{k!}x^k+...=\sum_{k=0}^\infty\frac{f^{(k)}(0)}{k!}x^k\]
\[f(x) = c_0 + c_1 x + c_2 x^2 + ...+ c_k x^k+...=\sum_{k=0}^\infty c_k x^k\]
When the center of the series moves to \(a\)
\[f(x)=f(a)+\frac{f^{'}(a)}{1!}(x-a)+...+\frac{f^{(k)}(a)}{k!}(x-a)^k+...=\sum_{k=0}^\infty\frac{f^{(k)}(a)}{k!}(x-a)^k\]
For Complex Function
\[f(z)=\sum_{n=0}^\infty a_n(z-z_0)^n,\;\left|z-z_0\right|<{R}\]
\[a_n=\frac{f^{(n)}(z_0)}{n!},\;n=0,1,2,...\]
Maclaurin series
Most Maclaurin series expressible in terms of elementary functions can be determined through the composition and combination of the following functions:
| Function | Maclaurin Series | Interval of Convergence |
|---|---|---|
| \(\dfrac{1}{1-x}\) | \(\displaystyle \sum_{k=0}^{\infty} x^k\) | \(-1 < x < 1\) |
| \(e^x\) | \(\displaystyle \sum_{k=0}^{\infty} \dfrac{x^k}{k!}\) | \(-\infty < x < \infty\) |
| \(\ln(1+x)\) | \(\displaystyle \sum_{k=1}^{\infty} \dfrac{(-1)^{k+1}x^k}{k}\) | \(-1 < x \leqslant 1\) |
| \(\sin x\) | \(\displaystyle \sum_{k=0}^{\infty} \dfrac{(-1)^k x^{2k+1}}{(2k+1)!}\) | \(-\infty < x < \infty\) |
| \(\cos x\) | \(\displaystyle \sum_{k=0}^{\infty} \dfrac{(-1)^k x^{2k}}{(2k)!}\) | \(-\infty < x < \infty\) |
Laurent series
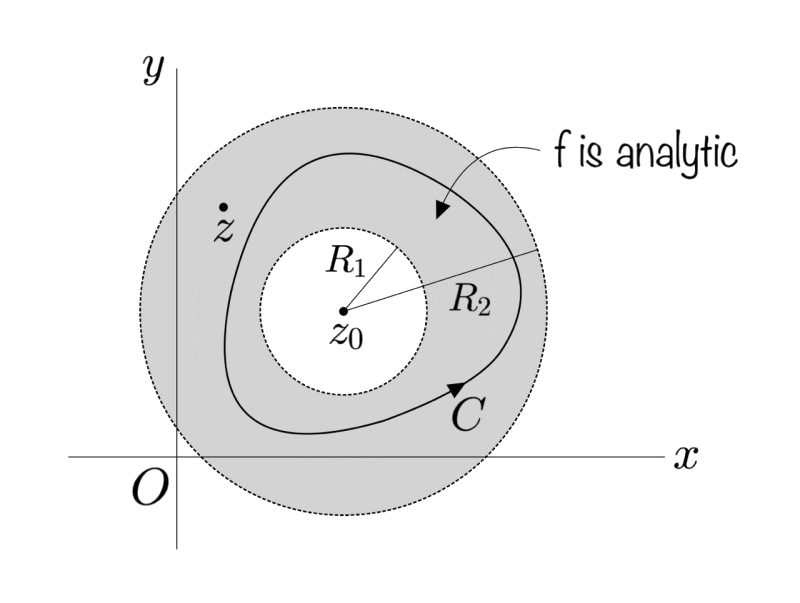
In the mathematics, the Laurent series of a complex function \(f(z)\) is a representation of that function as a power series which includes terms of negative degree.
Suppose that \(f(z)\) is analytic on the annulus
\[A: r_1 < |z - z_0|\;{<}\;r_2\]
Then \(f(z)\) can be expressed as a series
\[f(z)=\sum_{n=0}^\infty a_n(z-z_0)^n+\sum_{n=1}^\infty\frac{b_n}{(z-z_0)^n}\]
The coefficients have the formulus
\[a_n=\frac1{2\pi i}\oint_C\frac{f(z)dz}{(z-z_0)^{n+1}},\;n=0,\;1,\;2,\;...\]
\[b_n=\frac1{2\pi i}\oint_C(z-z_0)^{n-1}f(z)dz,\;n=1,\;2,\;3,\;...\]
where \(C\) is any circle \(|z-z_0|=r\) inside the annulus
Furthermore
The series \(\sum_{n = 0}^{\infty} a_n (z - z_0)^n\) converges to an analytic function for \(|z - z_0| {<} r_2\).
It is called the analytic or regular part of the Laurent series. [positive powers] (Taylor series)
The series \(\sum_{n = 1}^{\infty} \dfrac{b_n}{(z - z_0)^n}\) converges to an analytic function for \(|z - z_0| {>} r_1\).
It is called the singular or principal part of the Laurent series. [negative powers]
\[f(z)=...+\frac{a_{-m}}{{(z-z_0)}^m}+\frac{a_{-m+1}}{{(z-z_0)}^{m-1}}+...+\frac{a_{-2}}{{(z-z_0)}^2}+\frac{a_{-1}}{z-z_0}+a_0+a_1(z-z_0)+...\]
\[f(z)=\sum_{n=-\infty}^\infty a_n(z-z_0)^n\]
\[a_n=\frac1{2\mathrm{πi}}\oint_C\frac{f(z)}{{(z-z_0)}^{n+1}}dz,\;z_o\;is\;not\;at\;ROC\]
\[f(z)=\sum_{n=-\infty}^\infty(\frac1{2\mathrm{πi}}\oint_C\frac{f(w)}{{(w-z_0)}^{k+1}}dw)(z-z_0)^n\]
Cauchy's integral formula
\[f(a)=\frac1{2\mathrm{πi}}\oint_C\frac{f(z)}{z-a}dz\]
Ex
\(let\;C\;be\;the\;contour\;described\;by\;\left|z-1\right|=4,\;To\;find\;\oint_C\frac{e^{3z}}{z-\mathrm{πi}}dz\;around\;the\;contour\;C\)
\(f(a)=\frac1{2\mathrm{πi}}\oint_C\frac{f(z)}{z-a}dz\)
Sol
\(\oint_C\frac{f(z)}{z-a}dz=2\mathrm{πi}\cdot f(a)=2\mathrm{πi}e^{3\mathrm{πi}}\)
\(=2\mathrm{πi}(\cos3\mathrm\pi+\mathrm{isin}3\mathrm\pi)=-2\mathrm{πi}\)
Ex
To find \(\frac1{2\mathrm{πi}}\oint_C\frac{\mathrm{cosπ}z}{z^2-1}dz,\;C\;is\;the\;area\;(-2-i,\;2-i,\;2+i,-2+i)\)
Sol
\(\frac1{2\mathrm{πi}}\oint_C\frac{\mathrm{cosπ}z}{(z+1)(z-1)}dz=\frac1{2\mathrm{πi}}\cdot\frac12(\oint_C\frac{\mathrm{cosπ}z}{(z-1)}dz-\oint_C\frac{\mathrm{cosπ}z}{(z+1)}dz)\)
\(=\frac1{2\mathrm{πi}}\cdot\frac12\mathrm{cosπ}-\frac1{2\mathrm{πi}}\cdot\frac12\cos(-\mathrm\pi)=0\)
Pf
\(\oint_C\frac{f(z)}{z-a}dz=\oint_\Gamma\frac{f(z)}{z-a}dz\)
\(z=a+\varepsilon e^{i\theta}\)
\(dz=\varepsilon ie^{i\theta}d\theta\)
\(=\int_0^{2\mathrm\pi}\frac{f(a+\varepsilon e^{i\theta})}{\varepsilon e^{i\theta}}\varepsilon ie^{i\theta}d\theta\)
\(=i\int_0^{2\mathrm\pi}f(a+\varepsilon e^{i\theta})d\theta\)
\(\lim_{\varepsilon\rightarrow0}\oint_C\frac{f(z)}{z-a}dz=\lim_{\varepsilon\rightarrow0}i\int_0^{2\mathrm\pi}f(a+\varepsilon e^{i\theta})d\theta\)
\(=i\int_0^{2\mathrm\pi}f(a)d\theta\)
\(=2\mathrm{πi}\cdot\mathrm f(\mathrm a)\)
\(\therefore f(a)=\frac1{2\mathrm{πi}}\oint_C\frac{f(z)}{z-a}dz\)
Cauchy's differentiation formula
\[f^{(n)}(a)=\frac{n!}{2\mathrm{πi}}\oint_C\frac{f(z)}{{(z-a)}^{n+1}}dz\]
Ex
\(let\;C\;be\;the\;contour\;described\;by\;\left|z\right|=2,\;To\;find\;\oint_C\frac{e^{iz}}{z^3}dz\;around\;the\;contour\;C\)
\(f^{(n)}(a)=\frac{n!}{2\mathrm{πi}}\oint_C\frac{f(z)}{{(z-a)}^{n+1}}dz\)
\(\oint_C\frac{e^{iz}}{z^3}dz={\left.\frac{2\mathrm{πi}}{2!}f''(z)\right|}_{z=0}\)
\(=\frac{2\mathrm{πi}}{2!}\cdot i\cdot i\cdot{\left.e^{iz}\right|}_{z=0}=-\mathrm{πi}\)